Foil Calculator is a free online tool that shows how an equation can be simplified. However, our online foil calculator speeds up the process by displaying the simplification result in a matter of seconds. Meanwhile with our FOIL Method step-by-step calculator, you can get thorough answers to your arithmetic problems. However, with our math solver, you may practice your math skills and learn step by step.
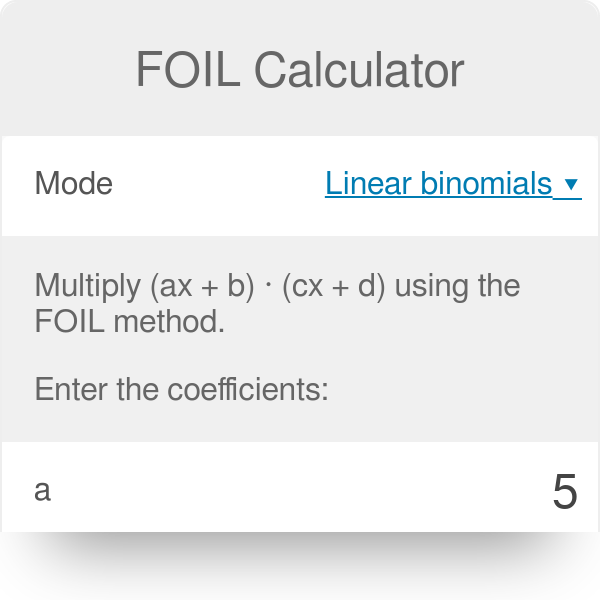
FOIL Calculator with steps
The following is how to use the foil calculator:
- Step 1:
Now in the input field, type the expression.
- Step 2:
Next, to receive the simplification, click the “Calculate” button.
- Step 3:
Finally, the output field will show the simplified expression.
FOIL Method Definition
It’s a method for distributing two binomials evenly. FOIL stands for First, Outer, Inner, and Last. However, the term “first” refers to multiplying the terms that appear first in each binomial. However, then multiply the product’s outermost terms with Outer. Thus, Inner refers to the innermost two terms being multiplied. Hence, last means multiplying the terms in each binomial that appear last. Then, however, to make the products easier to understand, merge any similar terms that may appear.
Example: (x + 2)(x + 5) = x·x + x·5 + 2·x + 2·5
First Outer Inner Last
= x2 + 7x + 10

However, the acronym FOIL was created as a mnemonic for high school algebra pupils. Meanwhile, the term first appears in William Betz’s Algebra for Today, published in 1929. However, William Betz was prominent in the American mathematics reform movement at the time, having published numerous texts on primary mathematical themes, and had “dedicated his life to the advancement of mathematics education.”
However, in the United States, many students and educators now use the term “FOIL” as a verb to imply “to extend the product of two binomials.”
Foil Method Meaning in Math
“First, Outer, Inner, Last” is the acronym meaning of FOIL. It is made up of the following elements:
- First (“first” terms of each binomial are multiplied together)
- Next, the first term of the first binomial and the second term of the second binomial are multiplied as “outside” terms.
- Inner terms (the second term of the first binomial and the first term of the second) are multiplied.
- Finally (“last” terms of each binomial are multiplied)
Example: (a+b)(c+d) = ac + ad + bc + bd
General form of FOIL method:

It’s worth noting that an is both a “first” and a “outer” term, and that b is both a “last” and a “inner” term, and so on. However, the sequence of the four terms in the total is unimportant, and the letters in the word FOIL do not have to be in the same order.
FOIL Method of Multiplication
FOIL stands for First, Outer, Inner, Last in mathematics. It’s a method for distributing binomials.
As an example, we can take (x+1) and (x+2). Both are binomials.
With the use of the FOIL method, it becomes (x+1) (x+2) = (x. x) + (x. 2) + (1. x) + (1.2)
FOIL method example with answer
(3z+5) (2z+7)
Using the FOIL approach, we can multiply the polynomials (3z+5) (2z+7). F O I L stands for multiplying the terms in each bracket in the order listed below: Outer by Outer (O O), Inner by Inner (I x I), and Last by Last (L x L): However, First by First (F × F), Outer by Outer (O × O), Inner by Inner (I x I), and Last by Last (L x L):
- (F × F) is (32) (2z)
- Next (0 x 0) is (3z)(7)
- (I x I) is (5) (2z)
- Then (Lx L) is (5) (7)
Then, combine the four terms in a sum:
(F XF) + (0 × O) + (1 × I) + (L × L):
Now, 3. 2z.z + 3.7z + 5.2z + 5.7
Multiply 3 times 2
6z .z + 3. 7z + 5.2z + 5.7
Multiply 3 times 7
6z.z + 21z + 5.2z + 5.7
Multiply 5 times 2
6z.z + 21z + 10z + 5.7
Multiply 5 times 7
6z.z + 21z + 10z + 35
Combining like terms 21z and 10z
6 z .z + 31z + 35
You can add the exponents when multiplying two powers with the same base (z).
62² +31z +35
Final Answer
6z²+31z +35
FOIL Method Binomials Calculator
A binomial is a polynomial with only two terms. When multiplying two binomials together, you can utilize the FOIL approach, which is simple to memorize. FOIL is an acronym for First, Outer, Inner, Last.
Sample problem:
(x+2) (x+1)
Step 1: Multiply each binomial’s first terms. However, the x from (x+2) and the x from (x+1) are the first terms here. Make a list of the products. (x2 is the product of x times x.)
Step 2: Next, in each of the two binomials, multiply the outer terms. However, the x from (x+2) and the 1 from (x+1) are the outside terms here. Make a list of the products. (The result of multiplying x by 1 is 1x, or x.)
Step 3: Multiply the two binomials’ inner terms. The 2 from (x+2) and the x from (x+1) are the inner terms here. Meanwhile, make a list of the products. (The product of two times x equals two times.)
Step 4: Multiply the final terms of the two binomials. The 2 from (x+2) and the 1 from (x+1) are the last terms. Meanwhile, make a list of the products. (The result of multiplying 1 by 2 is 2.)
You should have: x2 + x + 2x + 2
Step 5: Combine phrases that are similar. However, because there is nothing here with an x2, x2 remains unchanged, x and 2x can be joined to equal 3x, and 2 remains unchanged because there are no other constants.
Your final answer is: x2 + 3x + 2
FOIL Method Division Binomials
Example 1: (2x + 3)(3x – 1)
The proceeding steps indicate how to use FOIL on this multiplication question.
- Multiply each binomial’s initial term together.
(2x) (3x) = 6x²
- Multiply the phrases on the outside together.
(2x) (–1) = –2x
- Add the inner terms together to get the total.
(3) (3x) = 9x
- Multiply each expression’s last term together.
(3) (–1) = –3
- In order, list the four FOIL outcomes.
6x² – 2x + 9x – 3
- Combine the like terms.
6x² + 7x – 3
Example 2: (x – 3)(2x – 9)
See how the FOIL numbered steps work on a couple of negative terms.
- Multiply each binomial’s initial term together.
(x) (2x) = 2x²
- Multiply the phrases on the outside together.
(x)(–9) = –9x
- Add the inner terms together to get the total.
(–3)(2x) = –6x
- Multiply each expression’s last term together.
(–3)(–9) = 27
- List the four results of FOIL in order.
2x² – 9x – 6x + 27
- Combine the like terms.
2x² – 15x + 27
Example 3: [x + (y – 4)][3x + (2y + 1)]
This example is a little more involved, but FOIL makes things a lot simpler. However, the duties are broken down into smaller, more straightforward processes, and the outcomes are then merged.
- Multiply each binomial’s initial term together.
(x) (3x) = 3x²
- Multiply the phrases on the outside together.
(x)(2y + 1) = 2xy + x
- Add the inner terms together to get the total.
(y – 4)(3x) = 3xy – 12x
- Multiply each expression’s last term together.
However, two binomials make up the last terms. When you’ve completed this series of FOIL stages, you’ll FOIL these binomials.
(y – 4)(2y + 1)
- List the four results of FOIL in order.
3x² + 2xy + x + 3xy – 12x + (y – 4)(2y + 1)
- Combine like terms.
3x²+ 5xy – 11x + (y – 4)(2y + 1)
- FOIL the product of two binomials from Step 4: (y – 4)(2y + 1).
Multiple first term: (y) (2y) = 2y²
Multiply the outer terms: (y)(1) = y
Multiply the inner terms: (–4)(2y) = –8y
Multiply the last terms: (–4)(1) = –4
- Rewrite the issue by replacing the two binomials multiplied together with this new result.
3x² + 5xy – 11x + 2y² – 7y – 4
FOIL Method to Factor a Trinomial
You can use the FOIL method of factoring for polynomials with a non-prime leading coefficient and constant term (sometimes called the British Method). When factoring trinomials, the FOIL Method always works, and it’s a great tool to have if you can’t get your head around guess-and-check. When the FOIL approach fails, you may be positive that the quadratic in question is prime.
However, the FOIL technique of factoring requires you to reverse the procedures required for FOIL binomials. However, when you FOIL, keep in mind that you combine the first, outside, inside, and last terms. Then you combine any terms that are similar, which normally result from multiplying the outside and inner terms.
For example, to factor x² + 3x – 10, follow these steps:
● First, look for the Greatest Common Factor (GCF).
When you break down and examine the expression x² + 3x – 10, you’ll notice that it doesn’t have a GCF. The following is a breakdown:
( x . x ) + ( 3 . x ) – ( 2 . 5 )
Because no factors are shared by all terms, the expression does not have a GCF. You are now ready to go to the next step.
● Multiply the quadratic and constant terms together.
When performing this step, be cautious of the indicators. However, the quadratic term in this case is 1x², and the constant is –10, so
1x² . (-10) = -10x²
● Meanwhile, make a list of all the factors of the result that result in pairs, with an x for each term in each pair.
Now, –1x and 10x, 1x and –10x, –2x and 5x, and 2x and –5x are the pairings of –10x² factors in which each term contains an x.
● Find the pair that adds to produce the linear term’s coefficient from this list.
You ask the set whose total is +3x. For this question, the answer is –2x and 5x because
-2x . 5x = -10x²
And
–2x + 5x = 3x
● Divide the linear term into two terms, using the coefficients from Step 4 as a guide.
However, written out, you presently have x2 – 2x + 5x – 10.
● Divide the four terms into two groups of two each.
However, always put a ‘+’ sign between the two sets: (x2 – 2x) + (5x – 10).
● Find each set’s GCF and factor it out.
What are the similarities between the first two terms? a minus sign If you take the x out, you get x(x – 2). Meanwhile, take a look at the second and third terms now. However, they both have a 5. If you take the 5 out, you get 5(x – 2). x(x – 2) + 5(x – 2) is the new form of the polynomial.
● The GCF of the two new terms should be found.
As can be seen, (x – 2) appears in both terms, indicating that it is a GCF. However, factor the GCF to the front of both terms (it’s always the expression inside the parentheses) and leave the other terms inside the parenthesis. As a result, (x – 2)(x + 5) becomes (x – 2)(x + 5). After figuring out the GCF, the (x + 5) is what’s left (x-2).
Note: 1
In order to accurately factor out the GCF, the sign in Step 6 may need to change. However, if you don’t start with a plus sign between the two sets, you risk losing a negative sign that you’ll have to consider all the way through.
When factoring x² – 13x + 36, for example, you get the following polynomial in Step 5: x² – 9x – 4x + 36. (x² – 9x) + (– 4x + 36) is the result of grouping the terms. However, to get x(x – 9) + 4(–x + 9), factor out the x in the first set and the 4 in the second set.
Have you noticed how the second set is the polar opposite of the first? The sets must match exactly in order to proceed to the next phase. Replace the +4 in the middle with a –4 to get x(x – 9) – 4(x – 9). You can factor once more now that they match.
Note: 2
The FOIL approach works even if an equation has a leading coefficient other than 1. However, only if you can’t find any elements that add up to give you the linear coefficient in Step 2 does the monkey wrench appear. Meanwhile, the expression is prime in this circumstance. When you add the quadratic term of 2x² and the constant of 4 in 2x² + 13x + 4, you get 8x². However, no 8x² factors add up to 13x, making 2x² + 13x + 4 prime.
FOIL Method Distributive Property
The FOIL method is a two-step procedure that uses the distributive law.
(a + b) (c + d) = a(c + d) + b(c + d) + ac + ad + bc + bd.
The (c + d) is distributed over the addition in the first binomial in the first step. However, the distributive law is utilized in the second step to simplify each of the two terms. However, it’s worth noting that the distributive property is used three times in this operation. Unlike the FOIL approach, the distributive method can readily be applied to products with more terms, such as trinomials and higher.
FOIL Method Reverse
A product of two binomials is converted to a sum of four (or fewer, if like terms are joined) monomials using the FOIL rule. However, factoring, or factorization, is the opposite of factoring. The proof above, in particular, demonstrates the approach of factoring by grouping when read backwards.
Some Frequently Asked Questions about FOIL Method
What is the difference between FOIL and factoring?
However, when we multiply (x−3) times (x−4) to obtain x2−7x+12 x 2 − 7 x + 12 we call that operation “multiplying out” or sometimes FOILing. (FOIL stands for First, Outer, and Inner, Last, which is how we put the phrases together.) Factoring is the opposite of factoring.
How do you distribute 4 Binomials?
- Divide the first binomial into two halves.
- Next distribute each of the first binomial’s terms among the others.
- Then multiply the terms together.
- Simplify and merge any terms that are similar.
What is the FOIL method for factoring?
The FOIL technique of factoring requires you to reverse the procedures required for FOIL binomials. Meanwhile, when you FOIL, keep in mind that you combine the first, outside, inside, and last terms. Then, however, you combine any terms that are similar, which normally result from multiplying the outside and inner terms.
How do you Unfoil in math?
The procedure of factoring a trinomial into two binomials is known as unFOILing. However, when multiplying two binomials together, you utilize the FOIL method, which involves multiplying the First, Outer, Inner, and Last terms of the two binomials into a trinomial.